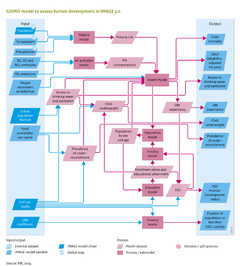
Human development/Description
Jump to navigation
Jump to search
Parts of Human development/Description
| Component is implemented in: |
|
| Related IMAGE components |
| Projects/Applications |
| Models/Databases |
| Key publications |
| References |